Seminario 18/25: Francisco Marcellán (Universidad Carlos III) A friendly approach to orthogonal polynomials and their applications

Información

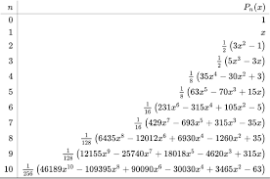
The theory of orthogonal polynomials constitutes a nice example of the interplay between classical analysis, numerical analysis, linear algebra, probability theory and mathematical physics. In this talk we will deal with orthogonal polynomials associated with measures

supported on the real line with a special emphasis on the situations where they are eigenfunctions of higher order linear differential operators. Analytic properties of such polynomials in a more general framework will be discussed.

Some applications in quadrature rules and boundary value problems will beanalyzed. The link with integrable systems (Toda lattices) will be shown. On the other hand, orthogonal polynomials with respect to nontrivial probability measures supported on the unit circle appear in the study of best linear predictors in filter theory. We will review some methods concerning the generation of such polynomials, their asymptotic behavior as well as the distribution of their zeros. Some applications to Szego quadratures and integrable systems, in particular Schur flows, will be presented.